This is from two Rudes I wrote nearly three years ago. I’ve combined them into one and hope it meets with your approval.

Feel free to write in with questions!

Seanie

Modern Portfolio Theory: Asset or Liability?

As you know, every summer, I teach recent college and MBA graduates who are entering banks.

It’s my job to teach total newbies about the financial markets and to get the business majors to be less theoretical and more practical.

You can probably tell that no one loves the sound of my voice more than I do. That’s why this will be my sixteenth summer teaching.

I can’t believe how fast it’s gone since March 2007, when I first started.

One of the critical areas for the kids - yes, they’re kids, and the darn things keep getting younger! - to learn is portfolio theory.

As banks are the “sell-side,” their clients are usually from the “buy-side.”

The buy-side consists of asset managers and hedge funds, who serve the “real money” clients.

Real money is the term we use to describe fully-funded, long-only asset managers and their clients: pension funds, sovereign wealth funds, insurance companies, and endowment funds.

That is, they have “real money” to invest and are not leveraged like hedge funds.

So, to know their clients, young bankers must learn what real money—or institutional investors, as they’re sometimes called—does with all the cash they have.

Modern Portfolio Theory: Background

One of the problems presented to fund managers is simple: what do we do with all this money?

In 1952, a young economist named Harry Markowitz wrote a paper called Portfolio Selection. It was published in The Journal of Finance, Vol. 7, No. 1 (Mar. 1952), on pages 77-91.

Markowitz studied at the University of Chicago under Milton Friedman.

In 1959, Markowitz published Portfolio Selection in expanded book form at the invitation of James Tobin, an eventual Nobel Prize-winning economist.

The Sveriges Riksbank Prize in Economic Sciences in Memory of Alfred Nobel 1990 was awarded jointly to Markowitz, Merton H. Miller, and William F. Sharpe "for their pioneering work in the theory of financial economics."

So one thing’s for sure: the theorists and prize winners love Modern Portfolio Theory. (That’s the name colloquially used to describe Markowitz’s entire body of work.)

The Purpose of Modern Portfolio Theory

I’m going to write as if I’m next to you with my coffee, so I’ll avoid jargon as much as possible.

The purpose of MPT is to maximize an investor’s returns for his given level of risk.

Markowitz confirmed that a portfolio’s return is the weighted average of the returns of the individual securities in a portfolio.

That is if stock A had an expected return of 2% and stock B had an expected return of 10%, the portfolio’s expected return would be (50% x 2%) + (50% x 10%) = 6%.

But Markowitz discovered that portfolio risk isn’t just a weighted average of the individual risks of the stocks. You had to take the correlation between the two stocks into account.

Without getting all mathy on you, let me visually demonstrate what happens with a portfolio of two stocks.

In our little sandbox, stock A has that 2% expected return and a 5% standard deviation. Stock B has that same 10% return with a 12% standard deviation.

They are the only two stocks we can invest in.

In finance, the standard deviation is synonymous with risk and volatility. This is based on a normal distribution or bell curve, and I’ll explain this more tomorrow.

 I will vary the correlation between the two stocks to show you how important it is.

In our first screenshot, both stocks are perfectly positively correlated. That is, they move in lockstep with each other.

If we’ve got a 50/50 portfolio, the expected return is 6%, the portfolio standard deviation is 8.5% (the average of the two stocks’ risks), and the minimum standard deviation we can achieve with this portfolio is putting 100% of our money in stock A, which has that 5% risk.

Then, it’s not much of a portfolio, is it?

What happens when there’s no correlation between the two stocks? That is, we can’t see a relationship between the movements of the two stocks at all.

What’s interesting about this is how the line is now a curve. If we stick with our 50/50 mix, we have a portfolio with the same expected return of 6% but an expected risk of 6.50%.

We lowered our risk by 2.00%, just by having assets that now did not correlate.

And if we wanted to minimize risk, we no longer had to put all our money in stock A. We would put 85% in stock A and 15% in stock B to achieve an expected portfolio risk of 4.62%.

That’s a much lower risk than when the stocks are perfectly positively correlated.

Let’s do one last example.

Let’s now make our stocks negatively correlated with each other. We won’t achieve a perfect negative correlation, but let’s make the correlation -0.70.

What happens?

With a -0.70 correlation, our 50/50 portfolio still has an expected return of 6% but a risk of only 4.61%. That’s a pretty dramatic reduction from 6.50%.

However, our minimum variance portfolio—the one with the lowest risk—only has a risk of 2.69%. We achieve that by investing 74% of our money in stock A and 26% in stock B.

To be sure, the entire curve is called the “minimum variance frontier.” The “efficient frontier” is the “top side of the bullet.”

That is, only those portfolios with a higher risk and a higher return than the minimum variance portfolio are what we’d call efficient.

That means they earn the highest return for a given level of risk.

MPT’s Assumptions, Finance’s La-La Land

Theorists make assumptions when they’re mathurbating.

Ahh, sorry. New word. Or portmanteau, to be specific.

The Urban Dictionary defines Mathurbation as

The art of substituting actually interesting content with complex-sounding but actually superficial math. Might bring some degree of arousal for the one performing it and some inexperienced and simple-minded spectators.

Example: Sannilkov's entire research has absolutely no contribution to science, it is just mathurbation.

Now that that’s out of the way, let’s discuss the assumptions Markowitz made to make the mathå work.

Asset returns are distributed normally.

Right off the bat, we have a ridiculous assumption. Sure, height, weight, and shoe size are normally distributed. But no returns anywhere in finance are normally distributed.

It’s little wonder why Nassim Taleb called the bell curve (or the standard normal distribution) The Great Intellectual Fraud in Fooled by Randomness.

The investor is rational and will avoid all unnecessary risks.

This one isn’t so bad, but it assumes the investor knows all the risks and can, thus, avoid them.

Investors will give their best to maximize returns for all the unique situations provided.

We know this simply doesn’t happen.

The largest investors rarely do this. Pension funds, insurance companies, sovereign wealth funds, and endowment funds routinely sell calls against their portfolios to smooth out their returns.

High net-worth individuals’ first priority is asset protection, not return maximization.

All investors have access to the same information.

Dearie me.

Sure, we can access stock data, charts, and news better than we ever could.

But do I get invited to dinner with Dalio, Fink, or Schwarzman?

No.

And if you don’t think dinner or golf matters, let me remind you Alan Greenspan made his “Irrational Exuberance” comments at the Annual Dinner and Francis Boyer Lecture of The American Enterprise Institute for Public Policy Research, in Washington, D.C., on December 5, 1996.

I woke up the next day wondering why the market tanked.

The people at the dinner doing the tanking clearly didn’t.

Investors don’t consider tax and trading costs when making decisions.

Taxes are most certainly taken into account in the States and other countries with a capital gains tax.

Where there’s no CGT, it’s obviously less of an issue.

Are trading costs accounted for now that it’s practically free? Probably not.

All the investors have the same view on the expected rate of return.

Again, I think not.

Sure, investors have similar views on the markets. But if we all had the same view, no trades would take place.

A lone investor is not sizeable enough to influence market prices.

BlackRock is. To be fair, most aren’t.

Investors can borrow unlimited capital at a risk-free rate.

Usually ridiculous, this feels awfully true at the moment.

In fact, so much capital is available to institutional investors that banks have to redeposit their excess reserves—the stuff they can’t loan out—at the Fed every day.

And they earn - their word, not mine - interest on it!

It’s about as preposterous as it gets, but that’s another conversation.

Should You Employ MPT?

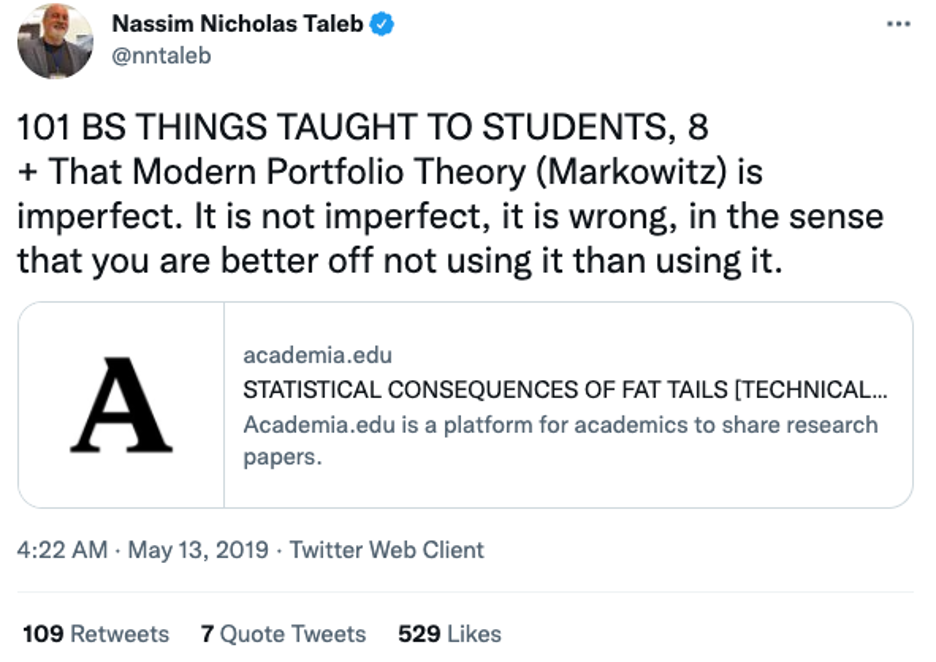
Ok, that’s not fair.

But Taleb further destroyed MPT in his book Antifragile - on my Rude Reading List - by alerting us to the fact that MPT relies on volatility and correlations remaining constant over time.

It’s plainly ridiculous. Here’s an excerpt:

I noticed as a trader— and obsessed over the idea— that correlations were never the same in different measurements. Unstable would be a mild word for them: 0.8 over a long period becomes −0.2 over another long period. A pure sucker game. At times of stress, correlations experience even more abrupt changes— without any reliable regularity, in spite of attempts to model “stress correlations.”

He goes on:

Note one fallacy promoted by Markowitz users: portfolio theory entices people to diversify, hence it is better than nothing. Wrong, you finance fools: it pushes them to optimize, hence overallocate. It does not drive people to take less risk based on diversification, but causes them to take more open positions owing to the perception of offsetting statistical properties…

Ok, that’s the man himself speaking.

What does that mean for you?

Buffett… or This Guy?

No one can be Warren Buffett.

Despite his folksy self-deprecation, there’s only one Warren Buffett. And there will only ever be one Warren Buffett.

Sure, read every one of his annual reports. It’ll make you a more thoughtful investor.

So while MPT is not necessary to follow, Buffett is out of everyone’s reach.

Now, let me introduce you to a man who has defied all investing trends, kept a low profile, and is respected by everyone.

His name is Tony Deden, and he’s the Chairman of Edelweiss Investments.

Tony was an early supporter of Ron Paul and is an avid follower of the Austrian School of Economics.

I met Tony years ago at a conference. Great guy - I had no idea who he was.

No, I don’t know him well. And he certainly wouldn’t remember me.

What separates Tony from the rest of the fund managers is his duty of care to his clients.

I found this fantastic blog post on thewoodshedd.com about Tony and his philosophy.

Reading it and understanding how he does business will make you do business better.

Heck, I wish I had found it sooner.

Tony rejected MPT long ago. You’ll see why in this post.

Please read the post after you’re done reading this. It’ll make you a little bit better today.

Thanks once again to Ed Kelly for his MPT request. I hope I did it justice.